(i) (cosec θ - cot θ)2 = (1-cos θ)/(1+cos θ)
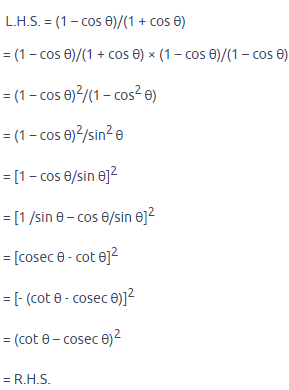
(ii) cos A/(1+sin A) + (1+sin A)/cos A = 2 sec A
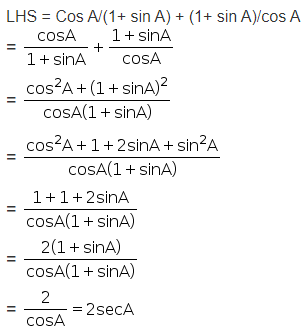
(iii) tan θ/(1-cot θ) + cot θ/(1-tan θ) = 1 + sec θ cosec θ
[Hint : Write the expression in terms of sin θ and cos θ]
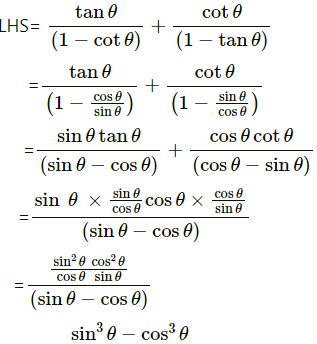
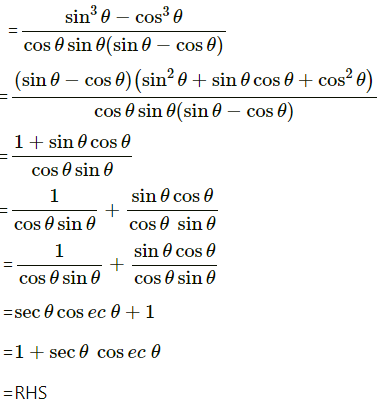
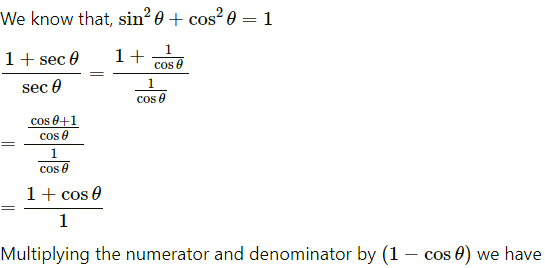
(iv) (1 + sec A)/sec A = sin2A/(1-cos A)
[Hint : Simplify LHS and RHS separately]
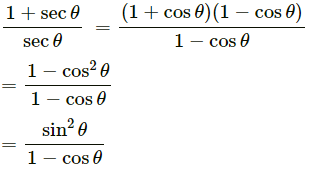
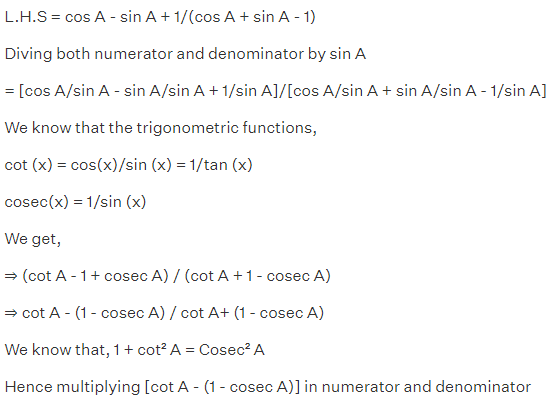
(v) (cos A–sin A+1)/(cos A+sin A–1) = cosec A + cot A,using the identity cosec2A = 1+cot2A

(vi) √1 + sin A/1 - sin A = sec A+ tan A
LHS = 1 + sin A/(1 - sin A) .....(1)
Multiplying and dividing by (1 + sin A)
⇒ (1 + sin A)(1 + sin A/1 - sin A)(1 + sin A)
= (1 + sin A)²/(1 - sin² A) [a² - b² = (a - b)(a + b)]
= (1 + sinA)/1 - sin² A
= 1 + sin A/cos² A
= 1 + sin A/cos A
= 1/cos A + sin A/cos A
= sec A + tan A
= R.H.S
(vii) (sin θ - 2sin3θ)/(2cos3θ-cos θ) = tan θ

(viii) (sin A + cosec A)2 + (cos A + sec A)2 = 7+tan2A+cot2A

(ix) (cosec A – sin A)(sec A – cos A) = 1/(tan A+cotA)
[Hint : Simplify LHS and RHS separately]

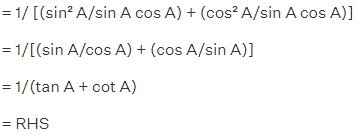
(x) (1+tan2A/1+cot2A) = (1-tan A/1-cot A)2 = tan2A













