Unitary Method

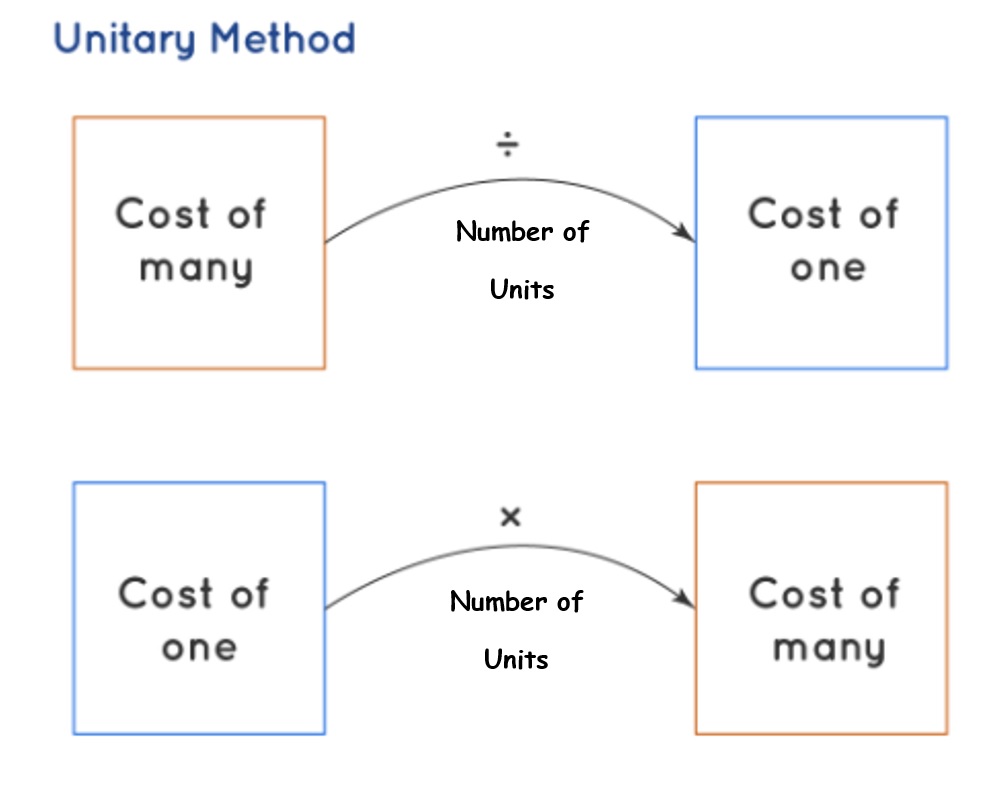
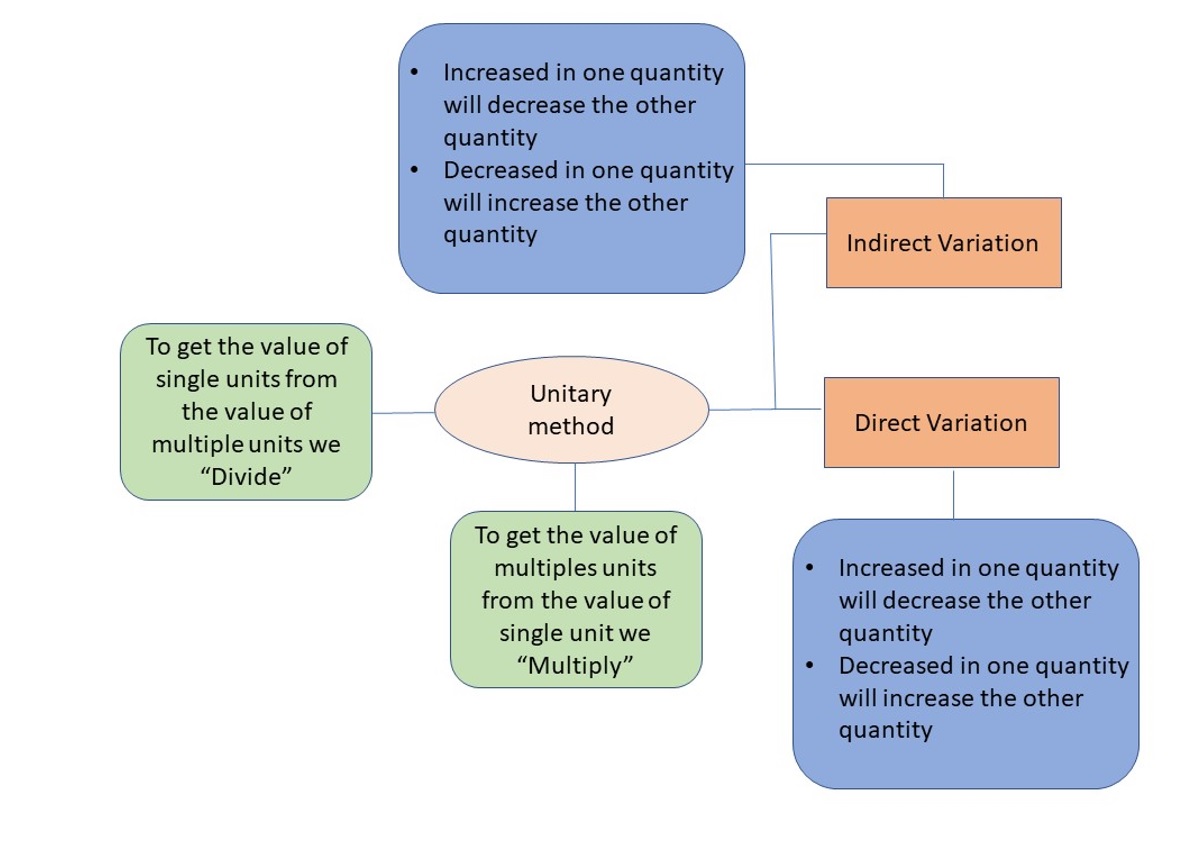
• Unitary method is defined the way to find the value of single unit from the value of multiple units or to find the value of multiple units from the value of single unit.
• The questions related to unitary method is solved using the concepts of “Multiplication” and “Division”.

Types of Unitary Method

The unitary method involved two types of the variations:

• Direct Variation
• Indirect Variation

Direct Variation:

It means that with the increase in one quantity the other quantity also increases.
For example:
If the number of articles increases then the cost will also increase.

Indirect Variation:

It means that with the:

Increase in one quantity, the other quantity decreases.
Decrease in one quantity, the other quantity increase.

For example: If the speed of the car increases then the fixed distance will be covered in less time, that is time will decrease.

Let us understand the how to use unitary method.

Method 1:

If the value of many units is given and we need to find the value of single unit then we divide the value of many units by the number of units.

Example:

The cost of 10 ice-creams is Rs 150. Find the cost of one ice-cream.

Solution:

The cost of 10 ice cream = Rs 150
Number of ice- creams = 10
Divide 150 by 10 to get the cost of 1 ice cream.
150 ÷ 10 = 15
Therefore, the cost of 1 ice cream = Rs 15.

Method 2:

If the value of single unit is given and we need to find the value of many units then we multiply the value of single unit by the number of units.

Example:

The cost of 1 pen is Rs 15. Find the cost of 5 pens.

Solution:

The cost of 1 pen = Rs 15
Number of pens = 5
Multiply 15 by 5 to get the cost of 5 pens.
15 × 5 = 75
Therefore, the cost of 5 pens = Rs 75.

Method 3:

If the value of more than one unit is given and we need to find the value of any number of units then we first find the value of the value of one unit and then the value of desired unit.

Example:

Cost of 10 eggs are Rs 60. Find the cost of 100 eggs.

Solution:

Given that, the cost of 10 eggs are Rs 60.
So, the cost of one egg is
60 ÷ 10 = 6
So, the price of one egg is Rs 6.
To find the price of 100 eggs we have to,
Multiply 100 by 6,
100 × 6 = 600
Therefore, the price of 100 eggs are Rs 600.

The general flow of unitary method is shown in the diagram below: