Addition and Subtraction of Fractions

 

When we talk about fractions, it's essential to understand the difference between like fractions and unlike fractions before learning how to add and subtract them.

What Are Like Fractions?

Like fractions are fractions that have the same denominator. For example:

• 2/5 and 3/5​ are like fractions because both have the denominator 5.

Unlike fractions are fractions that have different denominators. For example:

• 1/4 and 2/3 are unlike fractions because their denominators (4 and 3) are different.

How Do You Add Fractions?

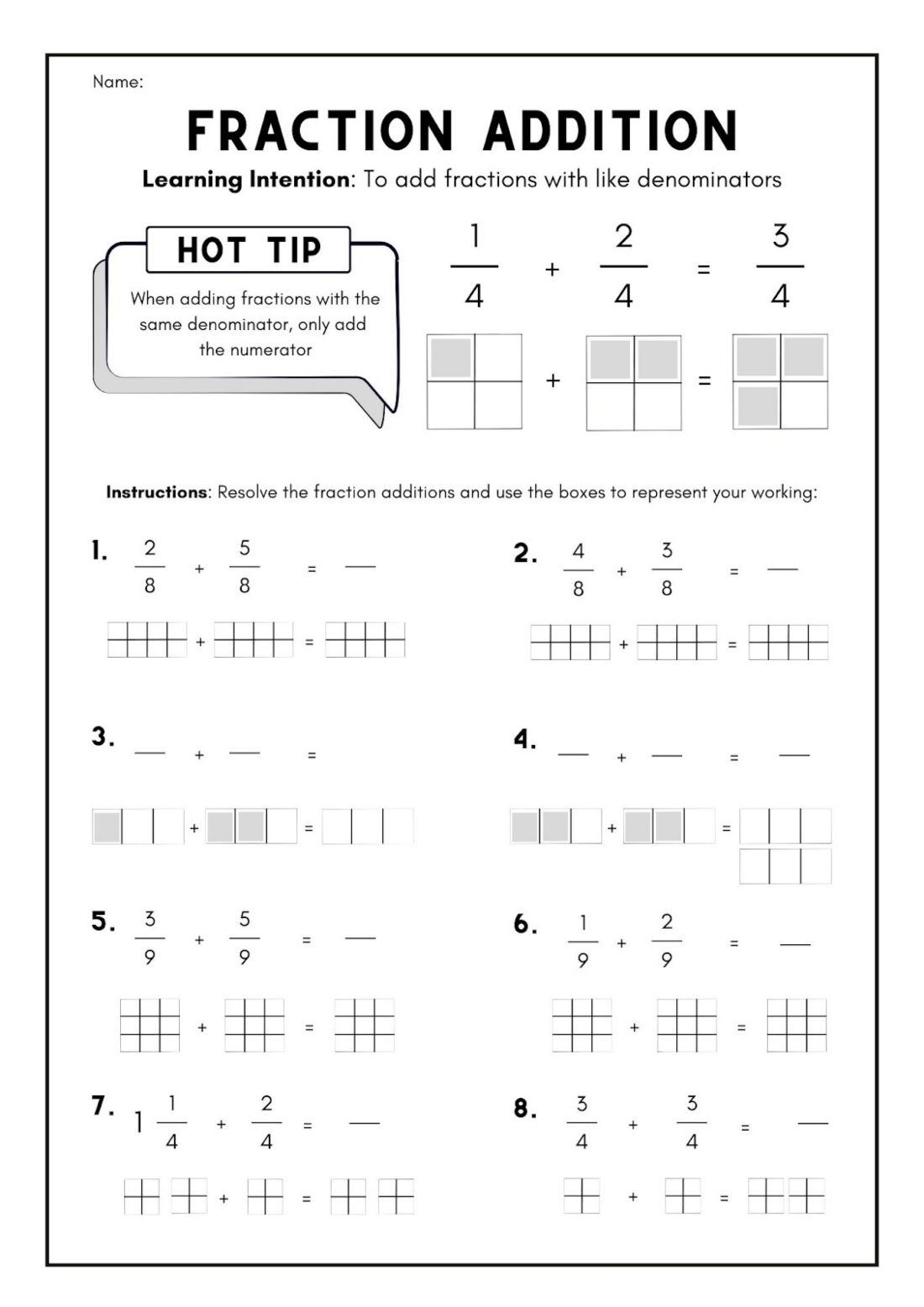
To add or subtract like fractions, you only need to add or subtract the numerators (the top numbers) and keep the denominator (the bottom number) the same.

Example of Addition of Like Fractions: 

2/7 + 3/7 = (2+3)/7 = 5/7

Example of Subtraction of Like Fractions :

5/8 - 3/8 = (5-3)/8 = 2/8 ( Simplify 2/8 to 1/4) 

Steps for Addition and Subtraction of Like Fractions:

1. Check the denominators: Make sure they are the same.

2. Add or subtract the numerators: Perform the operation (addition or subtraction) on the numerators.

3. Keep the denominator unchanged.

4. Simplify the fraction if necessary.

Like Fraction Example: 

ADD 1/6 and 4/6. 

Solution : 1/6 + 4/6 = (1+4) / 6 = 5/6

 

Interactive Quiz : 

Try these questions to practice what you've learned!

1. True or False: 3/5 and 4/5 are like fractions.

• True

• False

    2. Choose the correct answer: What is    2/7 + 4/7

•  6/7

• 8/7

• 6/14

3. Find the sum: 3/10 + 2/10 = ? 

4. Solve the subtraction: 7/9 - 3/9 =? 

5. Simplify the result: What is the simplified form of 8/12 - 2/12 ?  

• 6/12

• 1/2

• 3/4

Practice Questions

1. Addition Practice:  5/11 + 3/11

2. Subtraction Practice:  9 /15 - 4/15

3. Add the fractions: 1/4 + 2/4

4. Subtract and simplify: 10/14 - 4/14

5. Challenge Question:

• What is 3/8 + 4/8 - 2/8 ?  

Tips for Adding and Subtracting Like Fractions:

•  Always check if the answer can be simplified by dividing both the numerator and denominator by their greatest common factor (GCF).

• When adding and subtracting unlike fractions, first find a common denominator.

FAQs : 

 What is the rule for adding and subtracting like fractions?

To add or subtract fractions they must have the same denominator (the bottom value). If the denominators are already the same then it is just a matter of either adding or subtracting the numerators (the top value). If the denominators are different then a common denominator needs to be found.

 What is an example of subtraction of like fractions?

Step 1: Identify whether the given fractions have the same denominator or different denominators. 

Step 2: In the case of like fractions, subtract the numerators and write their difference over the common denominator. For example, 5/7 - 2/7 = (5 - 2)/7 = 3/7.

 What is addition and subtraction of like fraction?

It is very easy to add or subtract two or more like fractions. Follow the step given below for the addition and subtraction of like fractions: Step 1- Add or subtract the numerators of the fractions. Step 2- Write the result in the form of the numerator obtained in step-1 over the common denominator.

How do you subtract fractions with unlike denominators?

To subtract fractions with unlike denominators, use the least common denominator (LCD) of the two denominators:

• Find the least common denominator (LCD) of the denominators

• Rewrite each fraction using the least common denominator

• Subtract the numerators of the new fractions

• The denominator remains the same

Practice Worksheets

Click below for the practice worksheets...

Easy Level Worksheets

Intermediate Level Worksheets

Advance Level Worksheets